Write Your Answers In Trigonometric Form. Give Exact Values In
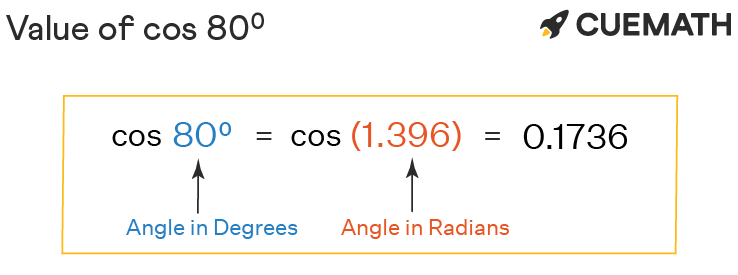
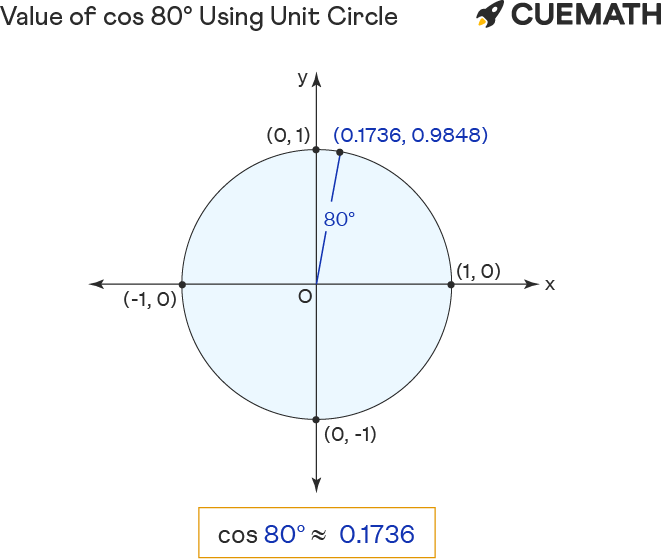
The cosine of 80 degrees can be calculated in both radians and degrees, with the value being 0.17365. To calculate in radians, you first select the desired unit and then enter the value in radians, which is 4/9π. The result can be shown in multiple forms, as an exact form or in decimal form. A cosine calculator can be used to easily calculate the cosine of any angle in radians. In the case of cos(80)°, the angle falls in Quadrant I and has a positive value for sin, cos, and tan. Using this simple cos calculator, the value of the cos of 80° can be calculated for both radians and degrees. In addition, to obtain 80 degrees in radians, you can multiply it by π/180° to get 4/9π. Finally, in the math problem, cos(20)cos(40)cos(80) is asked to be solved without a calculator.
For (z_1): (z_1 = 5(\cos 80^\circ + i \sin 80^\circ))
Using the trigonometric identity ( \cos \theta = \frac{e^{i\theta}+e^{-i\theta}}{2}) and ( \sin \theta = \frac{e^{i\theta}-e^{-i\theta}}{2i}), we can express (z_1) in the rectangular form:
(z_1 = 5\left(\frac{e^{i \cdot 80^\circ}+e^{-i \cdot 80^\circ}}{2}\right)) (z_1 = 5\left(\frac{\cos(80^\circ) + i \sin(80^\circ) + \cos(-80^\circ) + i \sin(-80^\circ)}{2}\right)) (z_1 = 5\left(\frac{\cos(80^\circ) + i \sin(80^\circ) + \cos(80^\circ) - i \sin(80^\circ)}{2}\right)) (z_1 = 5\left(\cos(80^\circ) + i \sin(80^\circ)\right))
Similarly, for (z_2): (z_2 = 10(\cos 140^\circ + i \sin 140^\circ)) (z_2 = 10\left(\frac{e^{i 140^\circ}+e^{-i 140^\circ}}{2}\right)) (z_2 = 10\left(\frac{\cos(140^\circ) + i \sin(140^\circ) + \cos(-140^\circ) + i \sin(-140^\circ)}{2}\right)) (z_2 = 10\left(\frac{\cos(140^\circ) + i \sin(140^\circ) + \cos(140^\circ) - i \sin(140^\circ)}{2}\right)) (z_2 = 10\left(\cos(140^\circ) + i \sin(140^\circ)\right))
To find (z_1/z_2): (z_1/z_2 = \frac{z_1}{z_2} = \frac{5(\cos 80^\circ + i \sin 80^\circ)}{10(\cos 140^\circ + i \sin 140^\circ)})
Dividing the magnitudes and subtracting the angles, we get: (z_1/z_2 = \frac{5}{10}(\cos(80^\circ-140^\circ) + i \sin(80^\circ-140^\circ))) (z_1/z_2 = \frac{1}{2}(\cos(-60^\circ) + i \sin(-60^\circ))) (z_1/z_2 = \frac{1}{2}\left(\frac{1}{2} - i \frac{\sqrt{3}}{2}\right))
Now, let's find (z_1z_2): (z_1z_2 = 5(\cos 80^\circ + i \sin 80^\circ) \times 10(\cos 140^\circ + i \sin 140^\circ))
Multiplying the magnitudes and adding the angles, we get: (z_1z_2 = 50(\cos(80^\circ+140^\circ) + i \sin(80^\circ+140^\circ))) (z_1z_2 = 50(\cos 220^\circ + i \sin 220^\circ))
So, the answers in the trigonometric form are: a) (z_1/z_2 = \frac{1}{2}\left(\cos(-60^\circ) + i \sin(-60^\circ)\right)) b) (z_1z_2 = 50(\cos 220^\circ + i \sin 220^\circ))
Work fast from anywhere
Stay up to date and move work forward with BrutusAI on macOS/iOS/web & android. Download the app today.