Why
The text outlines the steps for calculating the confidence interval. First, the standard error (SE) and margin of error (ME) must be calculated using a formula. The confidence level must then be set, usually at 90%, 95%, or 99%. A survey done on poverty levels in 1995 used a 90% confidence level. For a 90% confidence interval, the margin of error is 3.22 to 3.38. A similar calculation can be done for a 95% confidence interval using a different z value. Minitab can also be used to find multipliers. The process involves finding the sample mean, standard deviation, standard error, and margin of error, which can then be used in the formula to calculate the confidence interval. In this particular example, a 95% confidence interval is calculated for systolic blood pressure.
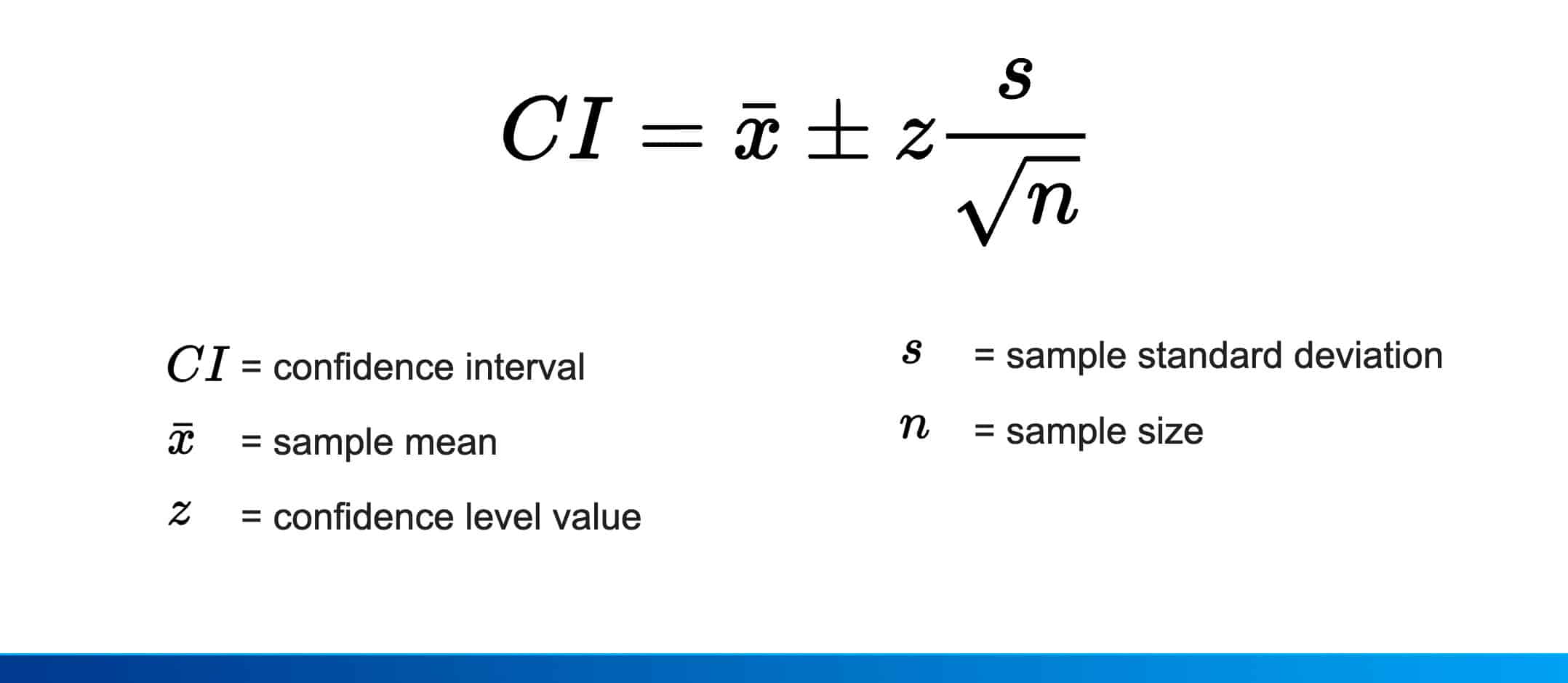
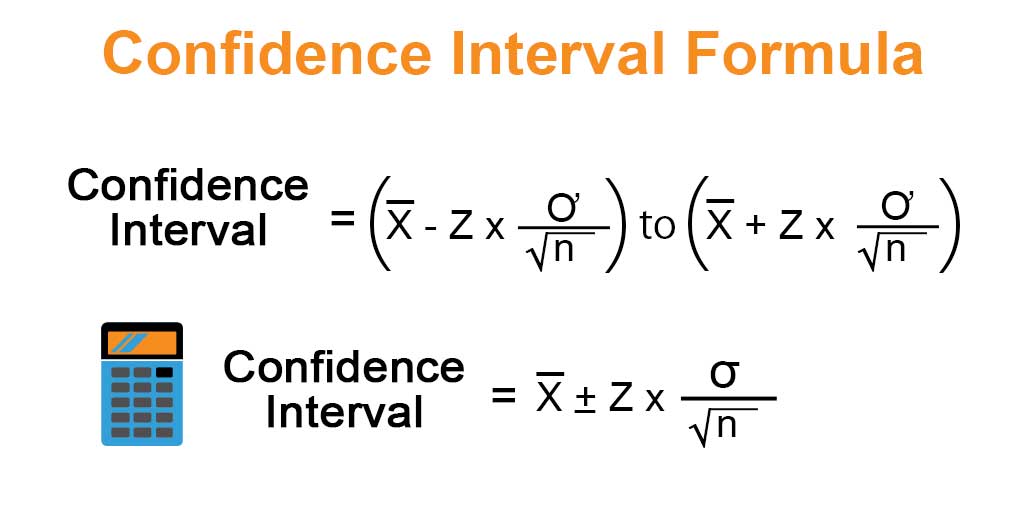
The correct method for calculating the confidence interval is using the formula:
[ \bar{x} \pm Z^* \left( \frac{s}{\sqrt{n}} \right) ]
In this case, the sample mean (( \bar{x} )) is 14.2, the Z-score for a 90% confidence level is approximately 1.645, the sample standard deviation ((s)) is 16.6, and the sample size ((n)) is 1014.
By substituting these values, we should get the 90% confidence interval for the mean number of books people read as 13.34 to 15.06 books. I'm unsure about the discrepancy. It seems there might be an error. Thank you for your understanding.
Work fast from anywhere
Stay up to date and move work forward with BrutusAI on macOS/iOS/web & android. Download the app today.