Suppose That The Distance Of Fly Balls Hit To The
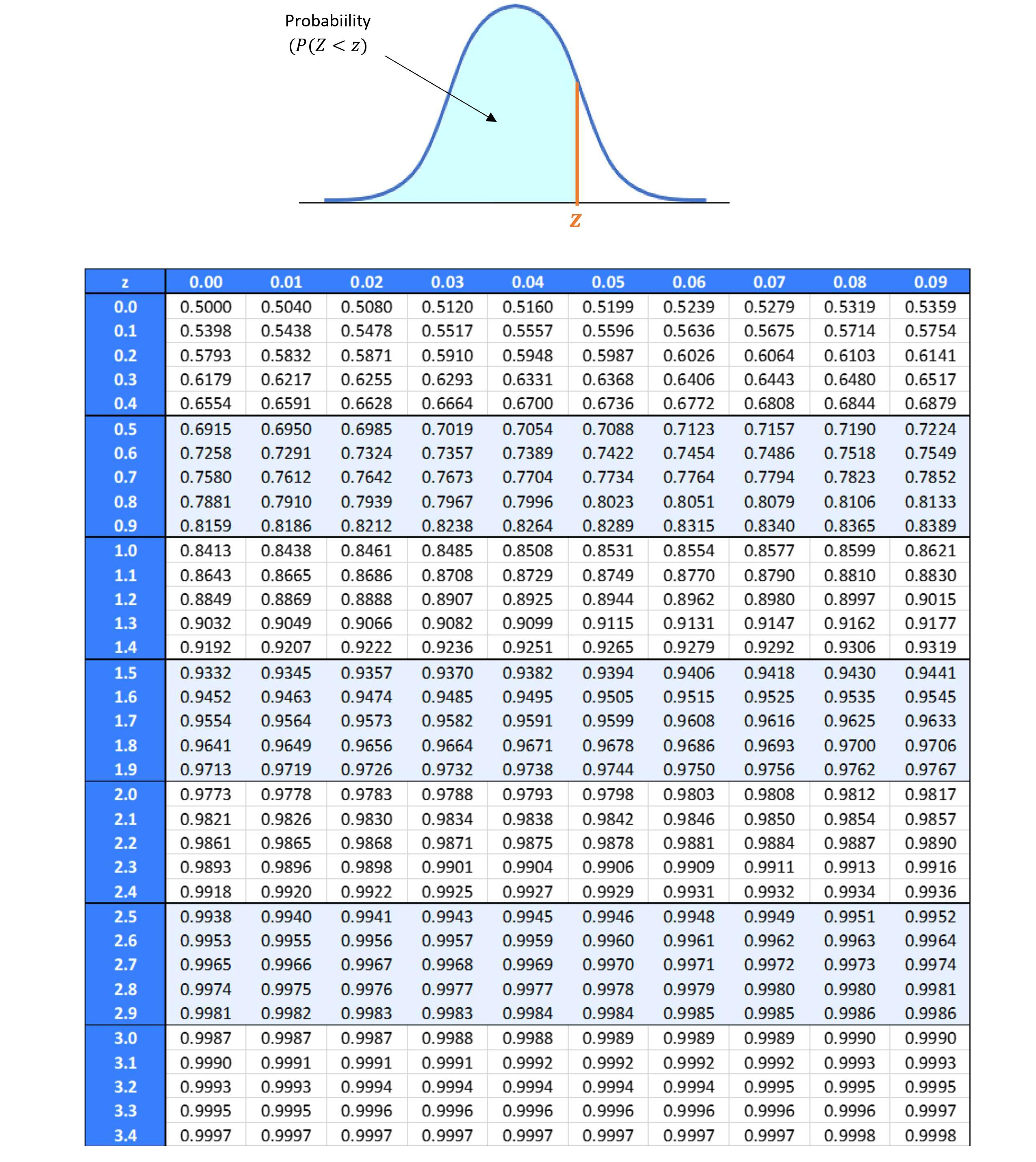
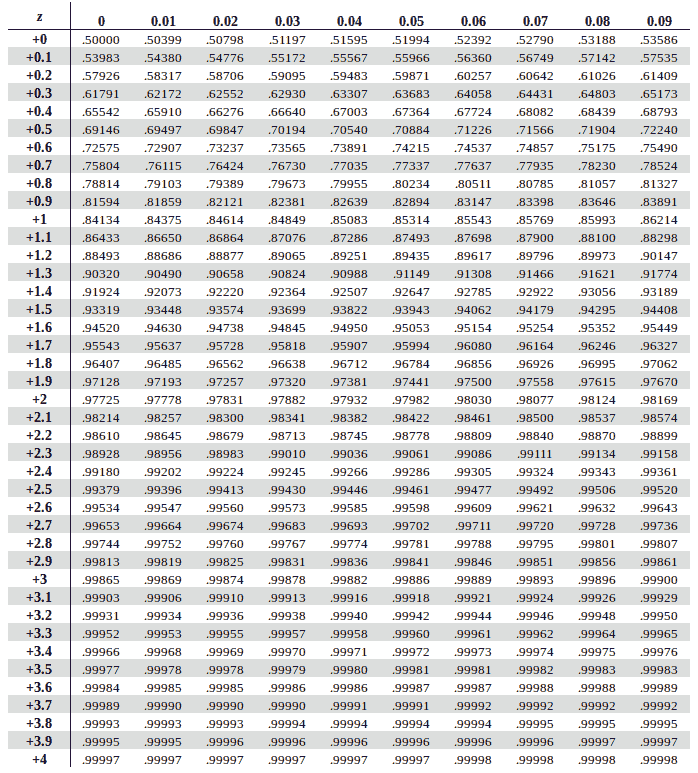
The text introduces the Standard Normal Distribution, a bell-shaped curve with a mean of 0 and standard deviation of 1. A table is provided with cumulative probabilities for positive z-values, and an additional table shows the cumulative probabilities for various z-values, including -3.4 and -3.9. These tables are also known as unit normal or z-score tables, and represent the area to the left of the z-score.
To solve this, we can use the Standard Normal Distribution. First, we need to standardize the sample mean using the formula z = (x - μ) / (σ / √n), where x is the sample mean, μ is the population mean, σ is the population standard deviation, and n is the sample size.
In this case: μ = 238 feet σ = 58 feet n = 49 x = 230 feet
Now we can calculate the z-score: z = (230 - 238) / (58 / √49) = -8 / (58 / 7) = -0.9655
Using the standard normal distribution table, we can find the probability that the sample mean is less than 230 feet by looking up the z-score -0.9655. The z-table provides the cumulative probability to the left of the z-score.
Looking up -0.9655 in the table, the probability is approximately 0.1660.
Therefore, the probability that the 49 balls traveled an average of less than 230 feet is approximately 0.1660, or 16.60%.
Sources
Related Questions
Work fast from anywhere
Stay up to date and move work forward with BrutusAI on macOS/iOS/web & android. Download the app today.
