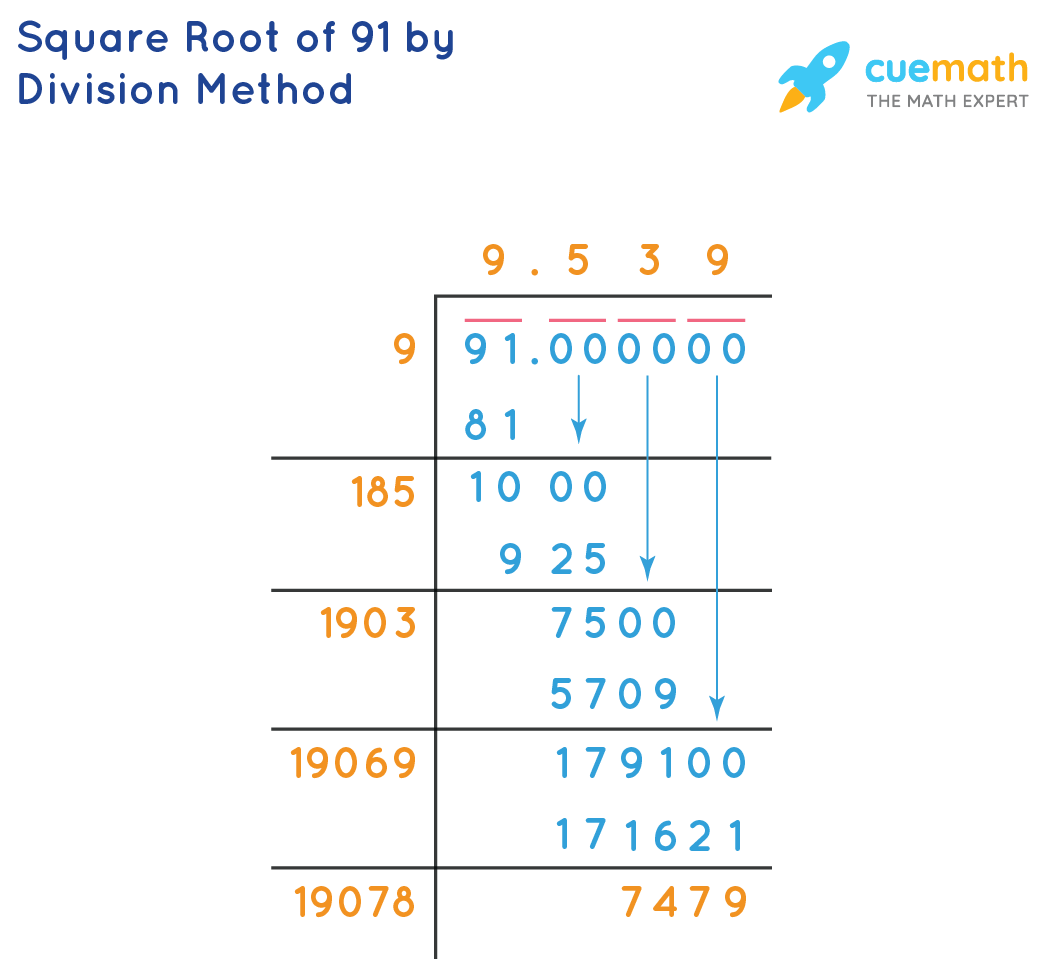Suppose Sin(𝜃) = 3/10 And Sec(𝜃) < 0. Find The ALugqFa
The text snippets focus on tools and methods for computing square roots and solving math problems involving fractions, rational numbers, and powers. These tools include a meticulously crafted calculator that can compute both positive and negative square roots, a free online calculator for finding square roots, a math problem solver with step-by-step explanations, and tips for rewriting fractions and integers.
We are given that sin(𝜃) = 3/10 and sec(𝜃) < 0.
From sin(𝜃) = 3/10, we can write cos(𝜃) = √(1 - sin²(𝜃)) = √(1 - (3/10)²) = √(1 - 9/100) = √(91/100).
Since sec(𝜃) = 1/cos(𝜃) and sec(𝜃) < 0, it means that cos(𝜃) < 0.
With these values, we can calculate sin(2𝜃) and cos(2𝜃).
First, we'll compute sin(2𝜃): sin(2𝜃) = 2 * sin(𝜃) * cos(𝜃) = 2 * (3/10) * (√91/10).
Now, we'll compute cos(2𝜃): cos(2𝜃) = cos²(𝜃) - sin²(𝜃) = (√91/10)² - (3/10)².
Let me calculate the values for sin(2𝜃) and cos(2𝜃) for you.
Work fast from anywhere
Stay up to date and move work forward with BrutusAI on macOS/iOS/web & android. Download the app today.