Suppose A Large Animal Has 2.75 Cm Thick Fur With 99cRGTf
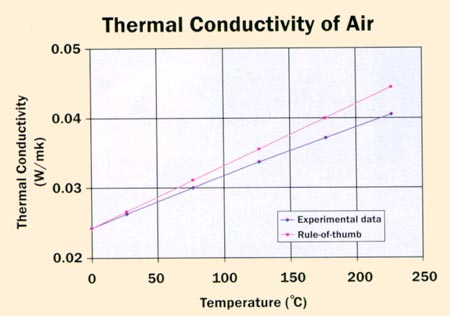
According to the text, thermal conductivity is an intensive property that measures a substance's ability to conduct heat. In heat transfer, it is represented by the letter "k" and is measured in units such as Btu(IT)/(ft h°F) or kcal/(m h°C). The thermal conductivity of air at 0°C is much lower compared to other substances, such as copper or carbon allotropes. The viscosity of air is dependent on temperature and ranges from 1.81 × 10-5 kg/(m·s) to 1.81 × 10-5 Pa·s at 15 °C. Lastly, air has a poor thermal conductivity of 2.623−6.763 × 10−2 W m−1 K−1 between 300 and 900 K.
The rate of heat conduction through the fur of the animal can be calculated using the formula:
[ \text{Rate of heat conduction} = k \times A \times \frac{T_s - T_a}{d} ]
Where:
- ( k ) is the thermal conductivity
- ( A ) is the surface area
- ( T_s ) is the skin temperature
- ( T_a ) is the air temperature
- ( d ) is the thickness of the fur
Given that the fur has the same thermal conductivity as air, and the thermal conductivity of air is in the range of 2.623−6.763 × 10−2 W m−1 K−1, we can use the average value.
Substituting the given values:
- ( k = 4.6935 × 10−2 , \text{W m}^{-1} , \text{K}^{-1} ) (average thermal conductivity of air at the given range)
- ( A = 1.15 , \text{m}^2 )
- ( T_s = 32.0°C )
- ( T_a = -5.00°C )
- ( d = 2.75 \times 10^{-2} , \text{m} )
Now, we can calculate the rate of heat conduction:
[ \text{Rate of heat conduction} = 4.6935 × 10−2 \times 1.15 \times (32.0 - (-5.00)) / 2.75 \times 10^{-2} ]
[ \text{Rate of heat conduction} \approx 944.57 , \text{W} ]
Therefore, the rate of heat conduction through the fur of the animal is approximately 944.57 watts.
Work fast from anywhere
Stay up to date and move work forward with BrutusAI on macOS/iOS/web & android. Download the app today.