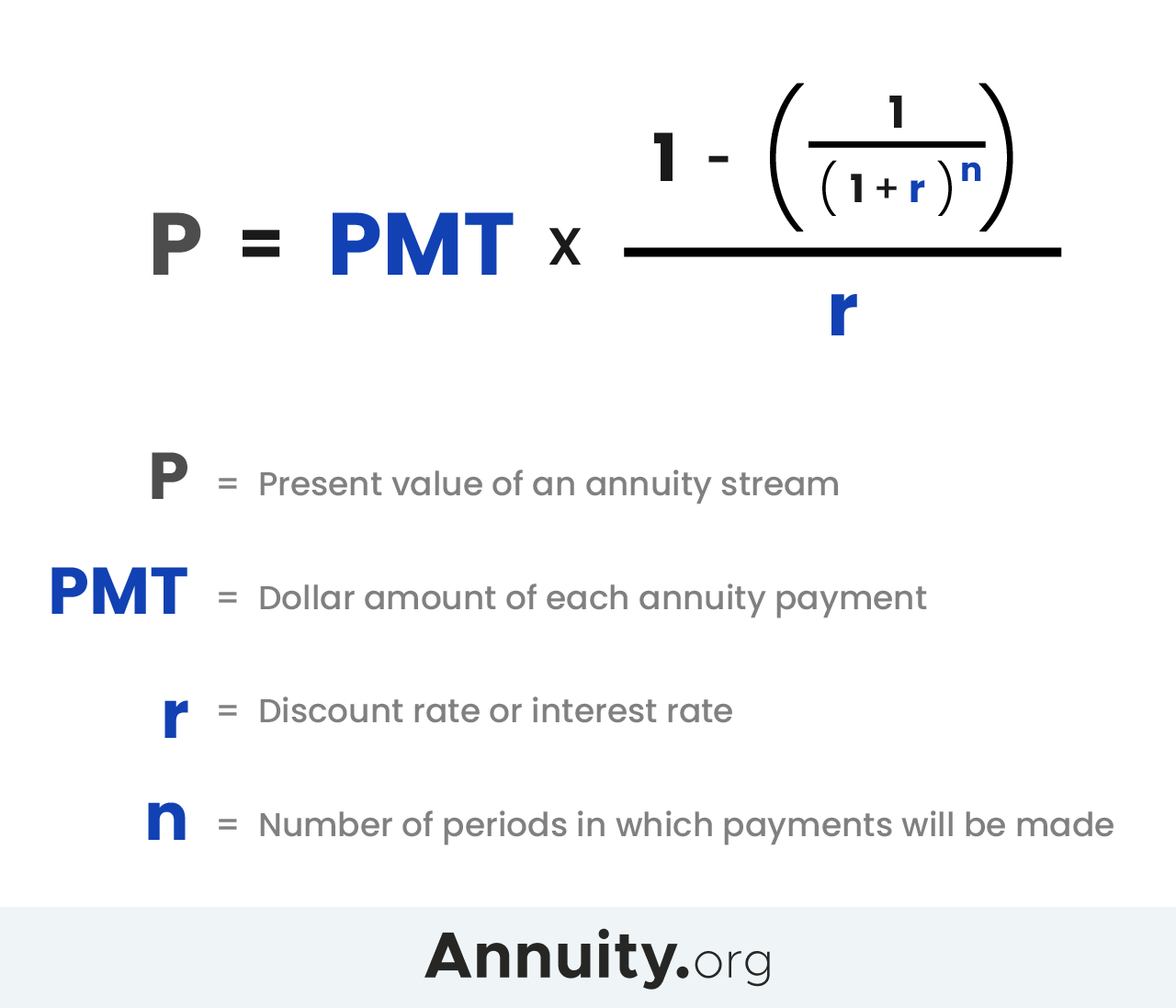
To calculate the present value (PV) of an annuity with the given information—an APR of 6%, monthly payments of $500, and a duration of 5 years—we can use the formula for the present value of an annuity:

[ PV = P \times \left( \frac{1 - (1 + r)^{-n}}{r} \right) ]

Where:

• (P) = monthly payment = $500
• (r) = monthly interest rate = ( \frac{0.06}{12} = 0.005)
• (n) = total number of payments = (5 \times 12 = 60)

Step-by-step Calculation:

1. Calculate ( (1 + r)^{-n} ): [ (1 + 0.005)^{-60} \approx (1.005)^{-60} \approx 0.740818 ]

2. Calculate ( 1 - (1 + r)^{-n} ): [ 1 - 0.740818 \approx 0.259182 ]

3. Compute the fraction: [ \frac{0.259182}{0.005} \approx 51.8364 ]

4. Finally, compute the present value: [ PV = 500 \times 51.8364 \approx 25918.20 ]

Result:

The present value of the annuity is approximately $25,918.20.

If you'd like to confirm this calculation using online tools, here are some useful links:

• Financial Mentor's Present Value of Annuity Calculator
• Calculator Soup's Present Value of Annuity Calculator
• Omni Calculator's Present Value of Annuity Calculator

Feel free to reach out if you have any further questions!

Sources

Present Value of Annuity Calculator - Financial MentorPresent Value of Annuity CalculatorPresent Value CalculatorAnnuity CalculatorAnnuity Calculator: Estimate Your Payout - BankratePresent Value of Annuity CalculatorPresent Value Calculator

Related Questions

What is the present value of a $300 annuity payment over 5 years if interest rates are 8 percent?

What is the present value of a $600 annuity payment over four years of interest rates are 6%?

How do you calculate the present value of an annuity payment?

What is the present value of an annuity if the interest rate is 5% per year for 5 years and the annual payments are $25000?