B. Construct A 95% Confidence Interval Estimate Of The Population
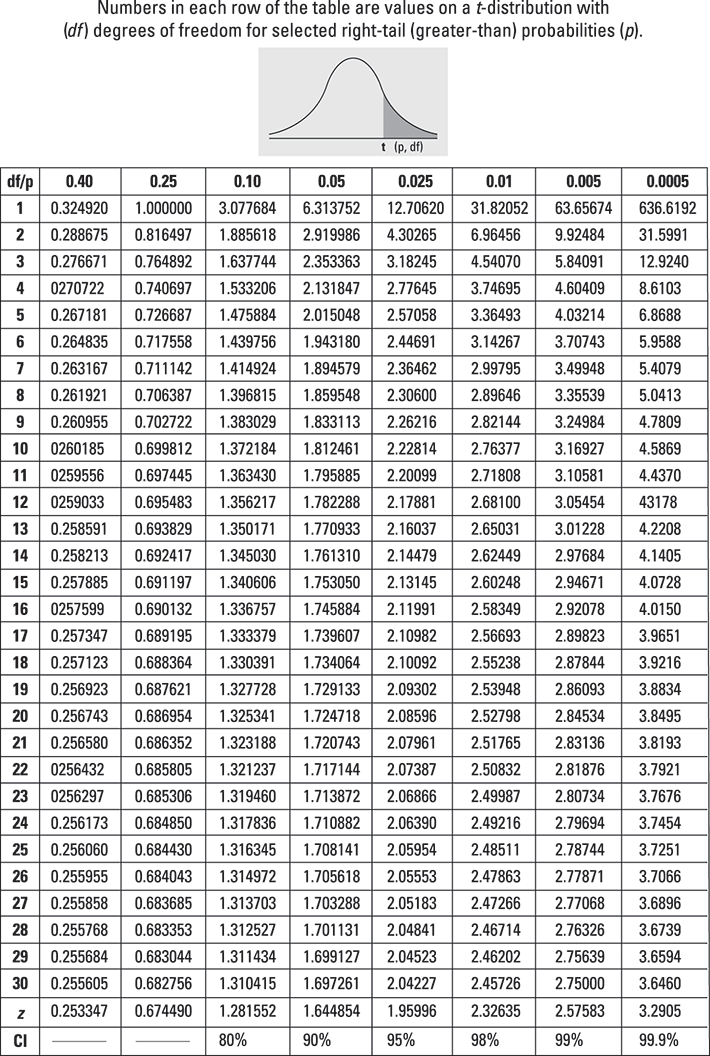
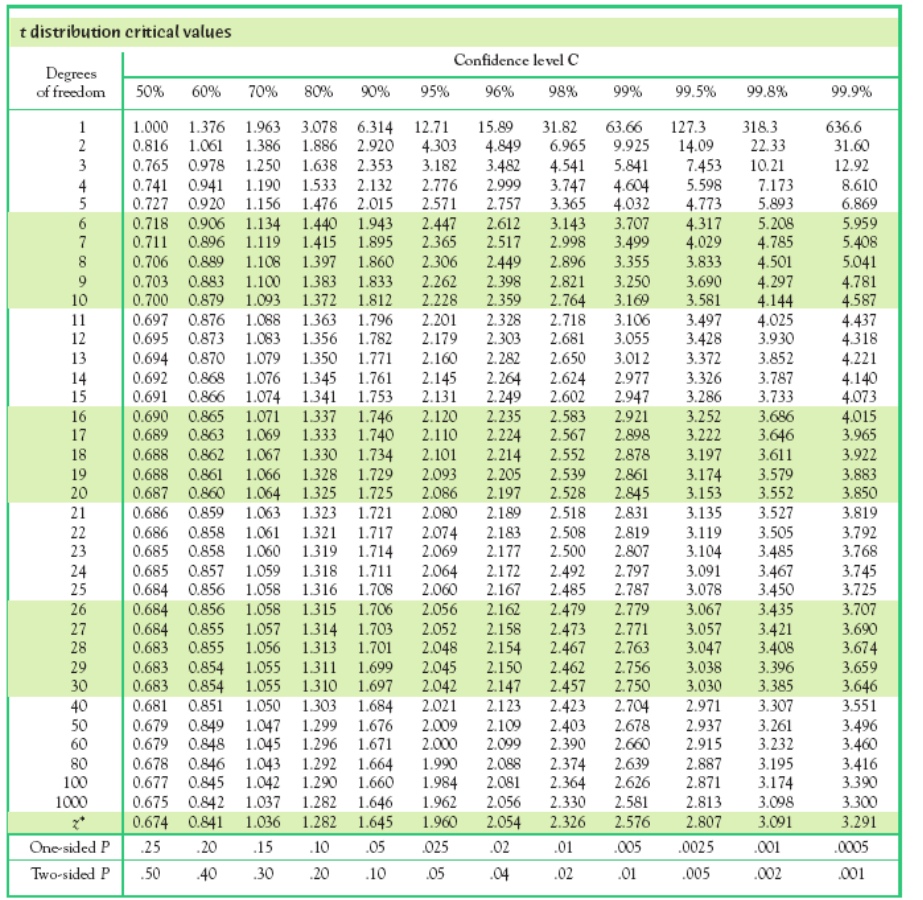
The text discusses the use of t-values and critical values in calculating confidence levels. These values are used to determine the level of significance for a particular statistic, and can vary depending on degrees of freedom and confidence interval. A t-table or calculator can be used to find the critical values for different degrees of freedom and confidence levels. Overall, these values are important in statistical analysis and can be helpful in determining the significance of a particular result.
The 95% confidence interval estimate of the population mean amount of tea per bag can be calculated using the formula:
[ \text{Confidence Interval} = \bar{x} \pm t^* \left( \frac{s}{\sqrt{n}} \right) ]
Where:
- ( \bar{x} ) = sample mean = 5.489
- ( s ) = sample standard deviation = 0.121
- ( n ) = sample size = 50
- ( t^* ) = critical t-value
The critical t-value for a 95% confidence level with 49 degrees of freedom is approximately 2.009 (obtained from a t-distribution table or calculator).
Substituting the values, we can calculate the 95% confidence interval estimate. Let's compute this.
Sources
Related Questions
Work fast from anywhere
Stay up to date and move work forward with BrutusAI on macOS/iOS/web & android. Download the app today.
