A Survey Of Nonprofit Organizations Showed That Online Fundraising Has Aa2P6yA
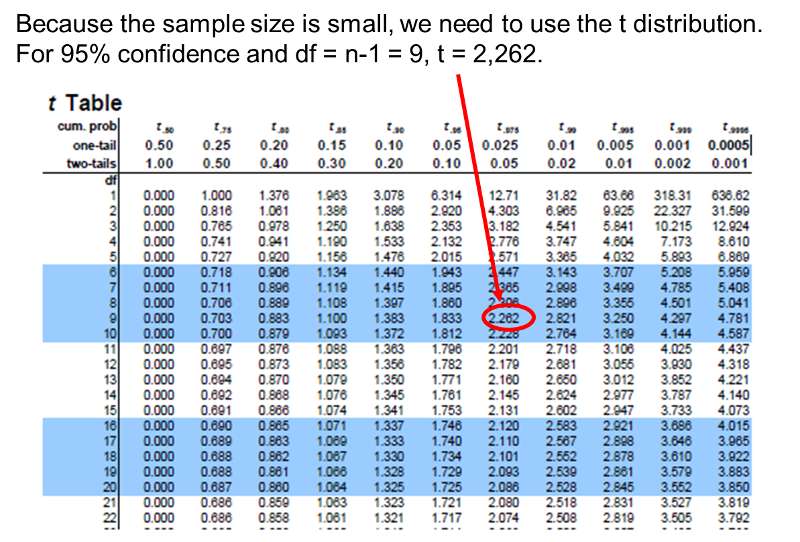
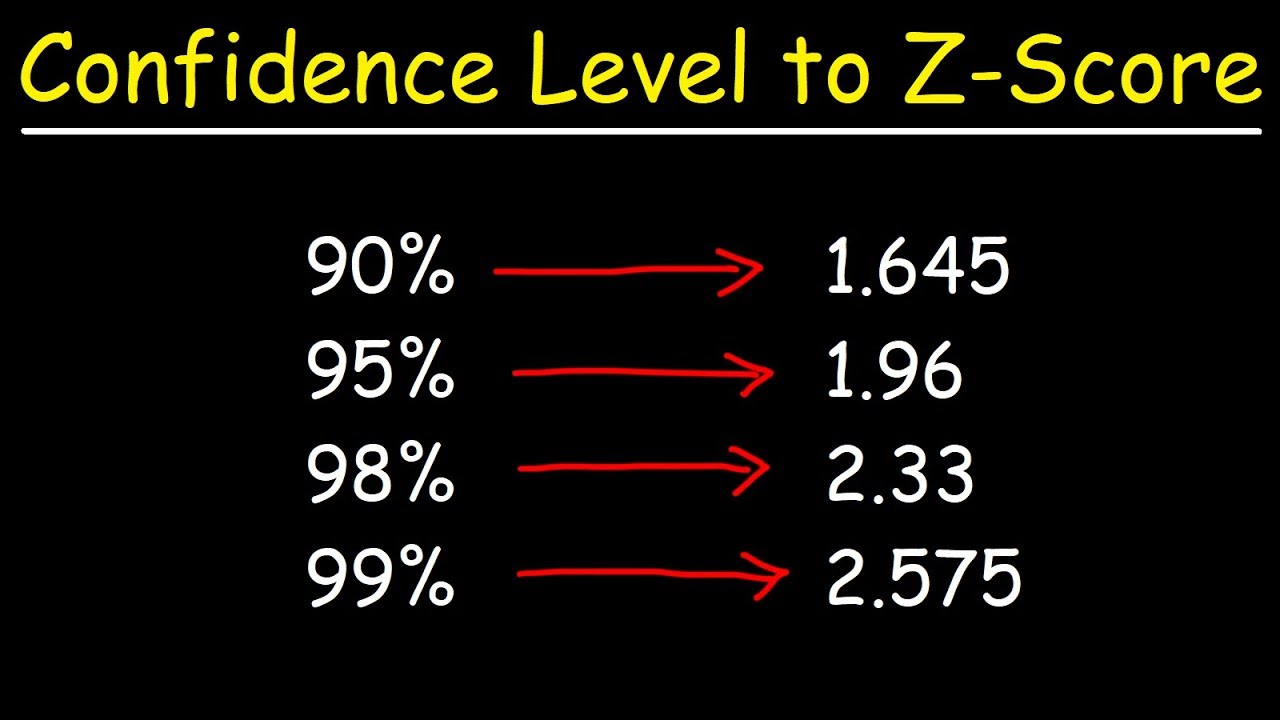
In statistics, a Z-Score, also known as a standard score, is a measure of how many standard deviations a data point is above or below the mean. A Z-Score can be used to calculate a confidence interval, with a Z-Score of 1.96 representing a 95% confidence level. Tables for Z-Scores and t-Scores can be accessed from external resources for further analysis. This concept is further explained in a video on statistics.
To construct a 95% confidence interval for the population one-time gift donation, we can use the formula:
[ \text{Confidence Interval} = \bar{x} \pm Z \left( \frac{s}{\sqrt{n}} \right) ]
Where:
- ( \bar{x} ) = sample mean = $29
- ( s ) = standard deviation = $7
- ( n ) = sample size = 50
- ( Z ) = Z-score for a 95% confidence interval, which is approximately 1.96
Substituting the values, we get:
[ \text{Confidence Interval} = 29 \pm 1.96 \left( \frac{7}{\sqrt{50}} \right) ]
Now, let's calculate this.
Work fast from anywhere
Stay up to date and move work forward with BrutusAI on macOS/iOS/web & android. Download the app today.