A) Construct An 80% Confidence Interval About Μ If The AG9QQHS
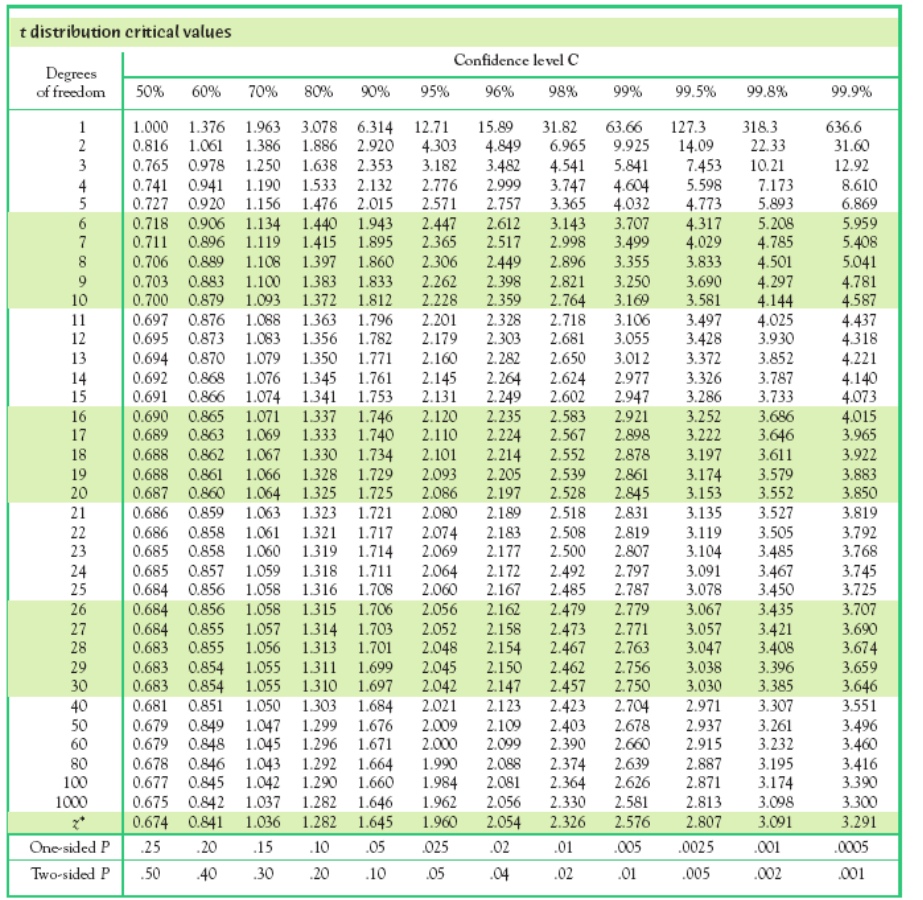
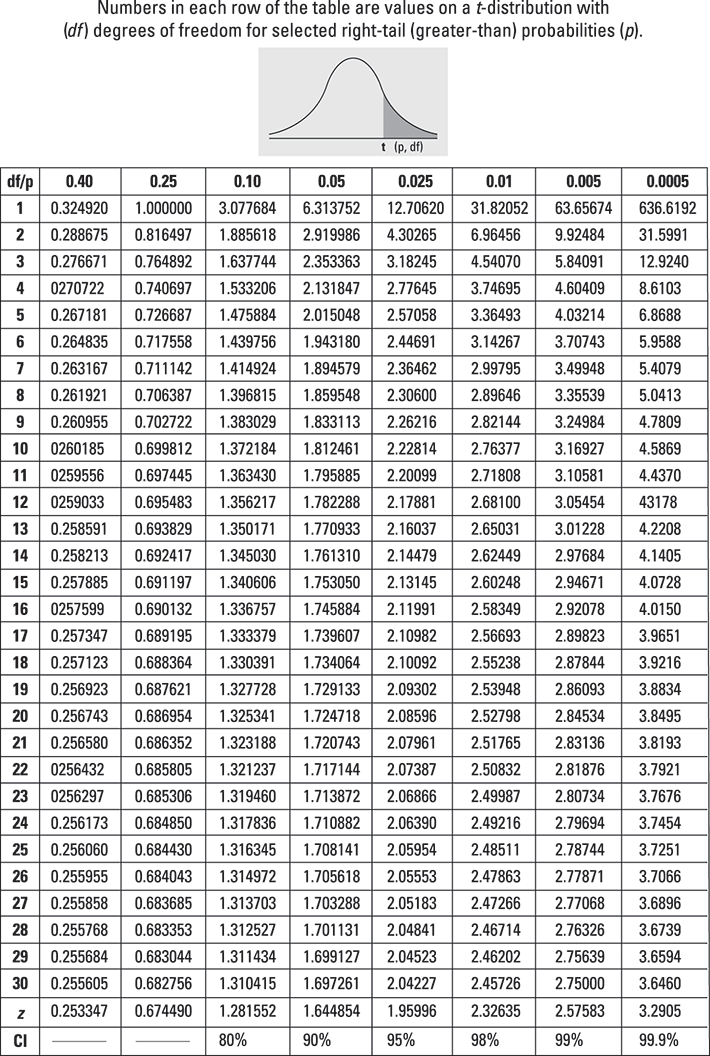
The text snippets provided are various t-distribution tables that are used for calculating critical values for different confidence levels and degrees of freedom. These tables provide the necessary information for conducting t-tests and calculating confidence intervals. The values in the tables correspond to different confidence levels and degrees of freedom, indicating the cutoff points for accepting or rejecting a null hypothesis in a t-test. These tables serve as a useful tool for statisticians and researchers in analyzing data and drawing conclusions.
For an 80% confidence interval with a sample size of 13, and using the t-distribution with 12 degrees of freedom, the critical t-value is approximately 1.782.
Using the formula for the confidence interval: [ \bar{x} \pm t^* \left( \frac{s}{\sqrt{n}} \right) ]
Where:
- (\bar{x}) = sample mean (108)
- (t^*) = 1.782 (from the t-distribution)
- (s) = sample standard deviation (10)
- (n) = sample size (13)
The margin of error (ME) is: (1.782 \times \frac{10}{\sqrt{13}} \approx 7.00)
For the confidence interval: Lower Bound: (108 - 7.00 \approx 101), Upper Bound: (108 + 7.00 \approx 115)
Therefore, the 80% confidence interval about (\mu) for a sample size of 13 is approximately 101 to 115.
Work fast from anywhere
Stay up to date and move work forward with BrutusAI on macOS/iOS/web & android. Download the app today.