Дві Кульки Однакової Маси ,рухаються Назустріч Зі Швидкостями 3 І
According to the text, there are three ways to solve elastic collision problems. The first way is to find the velocities of the two objects, v1 and v2, by using the conservation of momentum formula. The second way is to find the velocity of the center of mass by using the 22/11 formula. The third way is to use the formula for elastic collision, which involves masses, initial velocities, and kinetic energy. In order to solve an elastic collision problem, one must first identify the masses and velocities of the objects involved and their direction of travel before the collision, and then use the conservation of momentum and kinetic energy formulas to find the solution. This method can also be applied to head-on collisions between two particles, where their movements are considered.
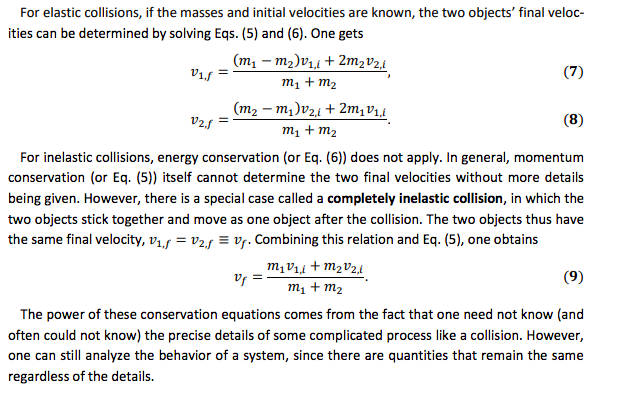
У випадку абсолютно упругого зіткнення маси рухомих тіл після зіткнення можна знайти наступним чином.
Закон збереження імпульсу стверджує, що сума імпульсів системи тіл до зіткнення дорівнює сумі імпульсів після зіткнення.
Нехай m - маса кульки 1, v1 - її початкова швидкість, m - маса кульки 2, v2 - її початкова швидкість.
Тоді сума імпульсів після зіткнення буде рівна сумі імпульсів до зіткнення: m1v1 + m2v2 = m1v1' + m2v2'
Отже, швидкість кульок після зіткнення визначається за формулою: v1' = ((v1*(m1-m2)) + 2m2v2) / (m1 + m2) v2' = ((v2*(m2-m1)) + 2m1v1) / (m1 + m2)
Після підстановки відомих значень мас і початкових швидкостей можна знайти швидкості кульок після зіткнення.
Sources

Related Questions
Work fast from anywhere
Stay up to date and move work forward with BrutusAI on macOS/iOS/web & android. Download the app today.
